List the Factors and Factor Pairs of a Whole Number

Students in the 5th grade should be able to list the factors and factor pairs of a whole number from 1-100 under Common Core standards.  For your convenience, the factors and factor pairs of whole numbers from 1-100 are included among the solutions provided by this application.  As the word factor suggests, a number is a part or factor of another number if it divides it without leaving a remainder, i.e., a remainder of 0.  In other words, a number is a factor of another number if it divides it evenly.  

For example, 4 is a factor of 24 because 24 divided by 4 equals 6 with a remainder of 0.  Similarly, 6 is a factor of 24 because 24 divided by 6 equals 4 with a remainder of 0.  Notably, 4 * 6 = 24.  As a result, (4 * 6) is one of several factor pairs of 24.  And from this example, we can see that factors and factor pairs of a number are byproducts of a multiplication table such as the one below. 

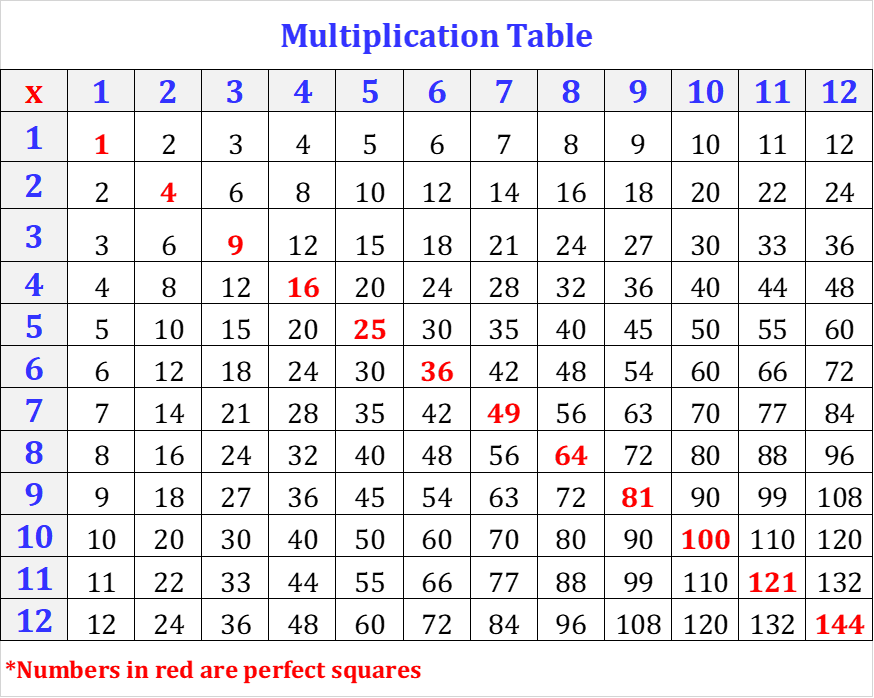
With respect to larger numbers that are not typically included in a multiplication table, it is helpful to know some divisibility rules in order to eliminate or reduce the number of possible factors that need to be checked.  Such rules include the divisibility by 3 rule. 

For example, 423 is divisible by 3 because the sum of the digits in 423 equals 9 (4+2+3) and 9 is divisible by 3.  As a result, 3 is a factor of 423 and 141 is also a factor of 423 since 423/3 equals 141.  Together, 3 and 141 form a factor pair of 423.  Notably, not all numbers that have a 3 as the last digit are divisible by 3 such as 43.  However, all whole numbers are divisible by 5 if the only digit or last digit is a 5 or 0 such as the case with 5 and 40.  And all even numbers are divisible by 2.  Furthermore, 1 and the whole number itself are always factors and they too form a factor pair.. 

One of the primary issues with finding all of the factors of a given number is deciding which numbers are possible factors and should be tested for divisibility.  In other words, we need a plan of attack other than guess and check. 

Notably, half of the factors of a given whole number are going to be less than or equal to its square root (divisor factors).  And dividing the given whole number by the divisor factors will produce the other half of the factors (quotient factors).  As a result, listing all the whole numbers (except 0) less than or equal to its square root as possible divisor factors is a recommended plan of attack. 

With exception of whole numbers that are also perfect squares, this method requires being able to estimate or determine the square root of a whole number based on its position between perfect squares.  For example, the square root of 45 is greater than 6, but it is less than 7 because 45 is between the perfect squares of 36 (6) and 49 (7).  Therefore, the list of possible divisor factors of 45 would be: 1, 2, 3, 4, 5, and 6.  Checking all of them for divisibility would guarantee finding all the factors and factor pairs of 45. 

As illustrated by the example of 146, the application above will find and list the factors and factor pairs of a whole number.   Take notice that 8 out of 12 possible divisor factors were filtered out by testing for divisibility.